World can be improved by simple action. Prism seat supports removed by three blows of a hammer. Seat lowered by 2 inches.
Did I notice the difference? I certainly did! Even two inches make a big difference!

In the preceding section, "The Prism", a one sheet boat concept was designed with the maximum displacement, or maximum volume in mind. Let's start something else now.
Any design process has the same basic phases:
Last time it was volume, something called "stability" didn't play a role. But, it obviously does play some kind of a role in boating, so let's redo the design, this time taking main criteria to be stability and boat looks. Keeping maximum volume in mind, however.
Remember, in the previous section the general idea was to build a single sheet boat with a straight middle part and tapered ends, cutting the plywood zig-zag in the middle. A nice length for the middle part turned to be 65", leaving 31" for the ends.
Three different cross sections were considered as giving the maximum volume: a semi circular with a radius of 15.3", a box with a bottom width of 24" and a dory shape with a bottom width of 16" and 60 degree side inclination. These will be the general starting points of the new "stability design".
A fourth hull cross section will also be considered now: a deep 90 degree V bottom. You know, a 2' x 2' box turned onto one corner. Why this? Because some of the following calculations were easiest on this cross section. I had to practise with something ;-)
But, on the other hand, the V hull is very simple, would be easy to make, so wouldn't it be nice if it were not too bad?
The analysis phase of a design involves prediction. Prediction is only possible, if You can make a model of what You predict. The model can be a scale model or a mathematical model, for example. Mathematical models are obviously nice and easy (once someone has created them).
Mathematical modelling for maximum volume is easy, because it is very clear what "volume" is and how to calculate a numeric value for volume. And with numeric values it is easy to tell what is more, what is less.
But what is "stability"? Guillemot kayaks have a nice introduction to small boat stability (read that first) on their pages. Their definition of stability is more or less "the ability of the boat to keep You out of water".
I like that definition a lot. But that definition does not help in judging which boat has more of that, which has less of that.
A detour might help.
Consider a spring, and a weight hanging from the spring. Pull on the weight, stretch the spring. The spring pulls on the weight, trying to return the weight to the equilibrium position. The spring pulls the harder, the stiffer the spring is. Let the weight go. The weight starts upwards, past the equilibrium, up, down, up, down, oscillating with a resonant frequency. The frequency is higher with stiffer springs and/or light weights. Add some friction, let the weight lean against a wall, for instance. The oscillation dies the sooner, the more friction. Enough friction, or damping - no oscillation.
There are torsional springs also. You don't pull a torsional spring, You twist or turn it. And weights attached to torsional springs don't oscillate up and down. Instead they rotate back and forth. The resonant frequency depends again on spring stiffness, but not on the weight mass. Instead, on the weight moment of inertia. Otherwise torsional springs and common "push-pull springs" behave similarly.
The torsion (T) excerted by the spring can be calculted by multiplying the so called "torsional spring constant" (K) (a measure of spring stiffness) by the angle the spring has been turned through (a).
T=-Ka
And the period (t) of the resonant oscillation (reciprocal of the resonant frequency) can be calculated from the moment of inertia (I) of the rotating weight and the mentioned torsional spring constant.
t=2*pi*sqroot(I/K)
It seems, that features of boat stability can be modelled using the torsional spring analogy model.
But before any modelling: Some items that probably contribut to small boat satbility:
Righting moment is the torque trying to return a boat into equilibrium, upright, when the boat is heeled.
This may be the most important single factor affecting the boat stability.
The magnitude of the torque can be calculated by multiplying the horizontal distance between the center of gravity of the boat and center of buoyancy of the underwater part of the boat by the boat displacement.
This sounds easy, but is not. To calculate the position of the center of buoyancy for all possible heeling angles and for all possible hull cross sections is not a trivial task.
Center of gravity sounds easier. You would think it lies on the center line of the boat, some distance above the bottom. This is more or less true for large boats. But for small boats, in the one sheeter or kayak category, it is not.
If the weight of the boat is 30 lbs and Your weight is 200 lbs, then the center of gravity of the boat plus You has very little to do with the boat, it's mostly about You. If You sit stiff in the middle of the boat, then the center of gravity is in line with the boat center line. But most of the time You don't sit stiff.
It seems, that when a small boat heels, most people "counter heel". If You model the center of gravity to lie on the boat centerline, the "counter heeling" has the same effect as lowering the center of gravity.
As long as the righting moment increases proportionally with the heeling angle, the boat acts like a theoretical torsional spring.
For decked boats (kayaks, sailing yachts, ships) it may be meaningful to consider boat stability up to 180 degrees heel (boat upside down), but for small open boats it is not.
Small open boats take water over the gunwale long before that. So in small boats the height of the boat side is what limits their stability in the end.
And in the one sheeter it's the width of the plywood sheet that limits the side height, along with the bottom width.
When a boat is heeled, then let go, or when an intermittent periodic force (such as waves) is applied to a boat, it starts to swing. This is where the spring model comes in handy.
The boat has a resonant frequency, governed by the spring constant from the righting moment and the moment of inertia. It is at this frequency the boat "wants" to swing. The reciprocal of this frequency is usually called "roll period".
Roll period is meaningful in at least two senses. First, it partly determines how comfortable You feel sitting in the boat. I suppose this has to do with how agile You are, how quickly You "counter balance".
Secondly, if the boat roll frequency coincides with the frequency of waves against the side of the boat, the energy from the waves feeds the boat oscillation. Depending on the roll damping (if it's too small) the boat swing amplitude may grow out of limits.
If the frequency of the waves is above the boat resonant roll frequency, the energy is not transferred. Small boats are meant only for small waves!
When a boat is heeled and then let go, the righting moment turns the boat up to the equilibrium position. If there is no damping, the boat comes up at speed, and has the greatest speed at zero degrees heel. The energy stored in the "spring" when heeled, has now been transformed to kinetic energy of the rotating boat hull.
Due to the speed the boat continues heeling to the other side, the kinetic energy being stored to the "spring" again. This is what the whole resonant oscillation is about. Transfer of energy between the spring and the rotating mass.
Unless some of the energy is taken away from the system.
Energy can be taken away by transferring it to the surrounding water. That is, the swinging boat hull must move water.
Flat and prodrucing shapes move more water than smooth shapes (like a paddle wheel). That's why semi circular boat shapes have less damping, box like boats more.
How much damping is needed? The question is the same as how stiff sould the shock absorbers under Your car be. Not too little, not too much. More is not necessarily better, definitely not the best.
Too stiff shock absorbers under Your car don't give suspension springs time to compress when the car hits a pot hole.
Similarly, too much boat roll damping doesn't allow the boat to heel quickly. Why should it? When water level is horizontal, You wouldn't want the boat to heel. But when water suddenly slopes, a passing power boat wave hitting You, for example, You would like to stay upright. And staying upright means the boat has to heel relative to the water surface, which is now suddenly sloping.
"Keeping You out of water" is not just about swinging the boat. If waves hit the boat directly from the bow the boat won't swing, but You still would like to have so much freeboard, that the wave crests don't fall over the gunwale.
The amount of sensible minimum initial freeboard depends at least on the boat size and boating conditions. In the one sheeter category I'd consider 6" as a minimum (with myself on board, that is).
If stability is "the ability of the boat to keep You out of water", it is really an on - off situation. Either You stay out of water, or You swim. So actually any boat, however tippy or rolling it may feel, is "stable" if You don't swim.
But if I interpret correctly, what most people mean when they say a boat is stable, is a boat with a high righting moment at low heeling angles and a lot of roll damping.
Real stability and perceived stability seem to be two different things. And they don't necessarily meet in the same boat.
This confuses me to such a degree, that this will be the last word "stability" I'll use in this section. From now on I'll try to stick to less vague terms. Note: I'm not using "democracy" nor "truth" either ;-)
OK. I want to try to design a one sheeter that is likely to keep You out of water. First, I want to calculate the righting moment curves for different hull shapes, different hull proportions and different heeling angles.
But mind You, I limit my calculations to single hull boat types. Twin hulls are perfectly possible even on a small boat, as can be seen on the ingenious Wavewalk boat. A twin hull would give yet another viewing angle to small boat stability.
"Why don't You use the Hulls software?" I hear You ask. Because "Hulls" puts the center of gravity at the center of boat cross sectional area. That may be a good first guess for larger boats (where most of the meaningful weight concentrates on the hull shell and possible deck). But now I want to be able to move the center of gravity at will.
So I worked out all sqaures and triangles and trigonometric functions to calculate the righting momets for four simple boat hull cross sections. And collected the stuff into a spreadsheet, to get a generic righting moment calculator.
Feel free to download the calculator form here as Excel 2000 calculator or here as Excel 4.0 calculator. They should work the same, the "2000" is the original. The calculator is bug free as far as I can tell (apart from fussiness at some angles... use 89.99 instead if 90 doesn't work... it's that simple).First of all, the bold numbers in the upper left hand side corner are the ones You can change. (Changing something else will probably screw the whole calculator ;-)
Displacement is clear, in a small boat it's the boat plus You. I've used 230 lbs for a single sheeter plus me.
It is assumed, that the boat is of the "Prism"
type, having a straight middle part. That's what
the "straight lenght" measure
is for.
The tapering ends contribute very little to the
righting moment, anyway. But what they contribute
comes as a bonus on top of what You get from
the calculator.
The height of the center of gravity above the bottom is just what it says. In a small boat a single passanger lying on the bottom would give a figure of 4", I guess. And sitting upright, 8" to 12" I'd guess. Guillemot kayaks say 10" is a standard guess.
The calculator gives You righting moment curves for four different hull cross sections:
In addition to the curves You get three numbers for each hull shape: the slope of the righting moment curve up to 10 degrees heel, the maximum righting moment and the work (or energy) needed to flood the boat. The work is proportional to the area under the righting moment curve.
The slope of the curve indicates how tippy the boat feels when it is about level, the other two give a hint of how difficult it actually is to flood the boat.
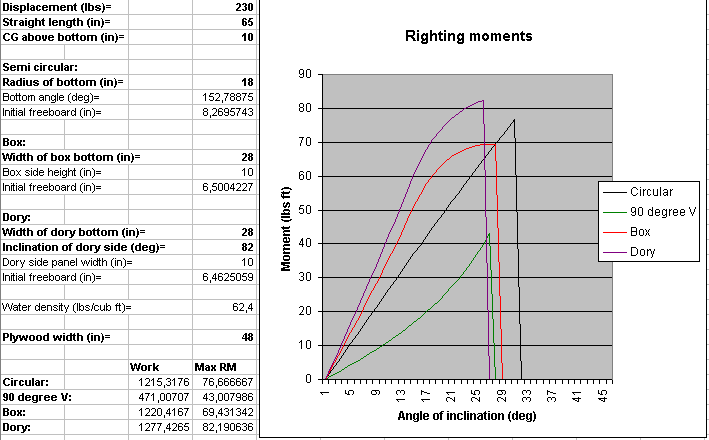
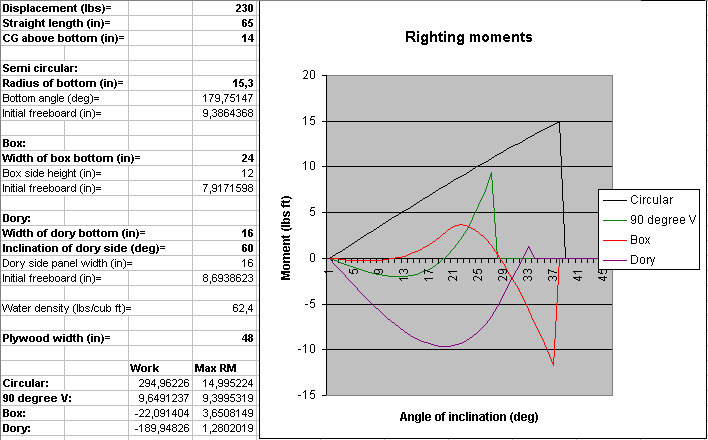
The "maximum volume" hulls from the previous section, with the center of gravity at the "standard" 10".
Surprisingly (or not?) the semi circular cross section is the best of all in all respects. It gives the greatest volume (remember from the earlier section), it gives the highest peak righting moment, it requires the greatest work to flood.
(Yes, of course I know a semi circular hull is not perfect, because it may roll like hell. But that's because of low damping, not because of low something else.)
The 90 degree V seems surprisingly good. The problem is, of course, that it is very difficult to get the centre of gravity low in a deep (thus narrow) V. But it might be ideal for transporting heavy, thin, straight objects. Lead pipes?
The 60 degree dory is flippy near zero inclination, but stiffens when heeled. This is supported by the experience from the Prism. Looking at it another way: this curve describes a usable boat. Anything above it - perhaps more usable.
The maximum volume box shows what happens when the opposite chine raises out of water. The maths change suddenly and there is a marked change in the righting moment curve.
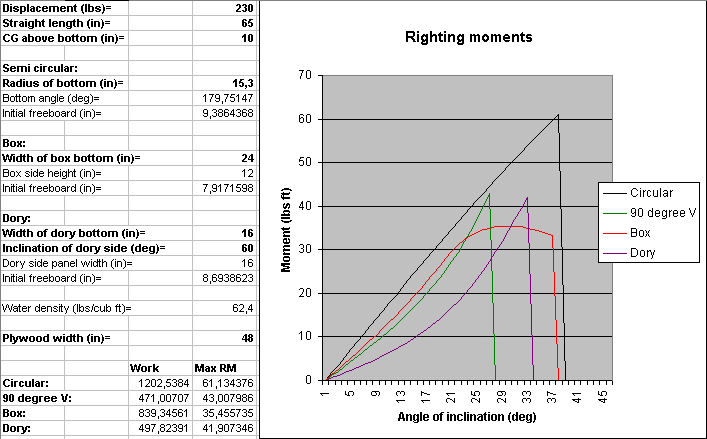
Exactly the same boats, but center of gravity at 4". The change is almost unbelievable!
All righting moments at least double. The energy to flood the dory shape triples! And all the curves now describe nearly ideal, surpriseless springs.
Had I understood this at the time I built the Prism I would have left the seat out. The seat surface is 75 mm (3") above the bottom. Removing the seat would lower the center of gravity by 3". I quess I'll try to remove the seat.
Moral of the story: Put Your seats as low as possible. At the cost of sitting in bilge water ;-(
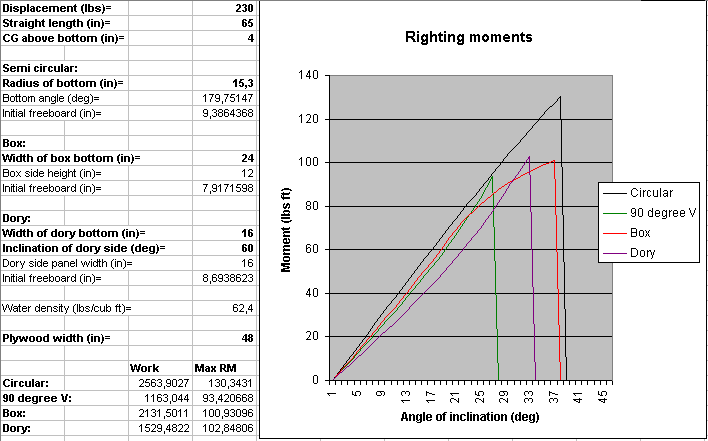
But lift the center of gravity to 14". All boats turn into catastrophes.
Moral of the story strengthened: Put Your seats as low as possible.
Second moral: Roll period calculations are meaningless for small boats. Remember from above: roll period is proportional to the square root of moment of inertia divided by spring constant. And it now seems it's not the hull shape but the height of the centre of gravity that determines the spring constant (the slope of the righting moment curve).
And as the moment of inertia goes: mass near the roation axis - small inertia, mass far from the axis - large inertia.
Combined: I lie on the bottom of the boat, my moment of inertia about the boat axis is low. At the same time I put the center of gravity low, follows a high righting moment, a high moment curve slope, a high spring constant. Low inertia divided by high spring constant - quick roll period. Regardless of the hull shape.
I sit in the boat, resulting in a higher moment of inertia, but at the same time low righting moment. Resulting in a slower roll period. Regardless of the hull shape.
And remember, damping is another thing.
 |
World can be improved by simple action. Prism seat supports removed by three blows of a hammer. Seat lowered by 2 inches. Did I notice the difference? I certainly did! Even two inches make a big difference! |
OK. Then try to find optimum hull shapes as far as righting moment is concerned. There are two different cases. Should one look for maximum righting moment or for maximum work to flood? I don't know, I'd suppose both are important.
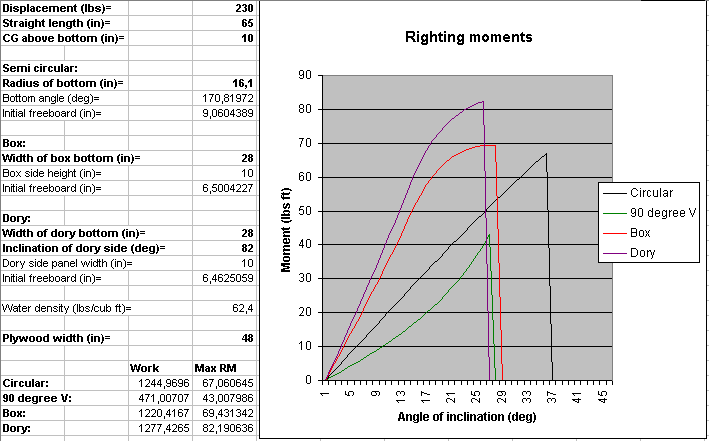
So let's look for maximum work to flood first. By playing around for a while I ended up at these shapes. (Note again, the V-shape can not be changed, so in every graph it represents the same boat. Unless the displacement, length or ply width are changed.)
The best result is given by a dory shape with 28" bottom and 10" sides inclined at 82 degrees. Oh hell, that's not a "dory", that's a box. The top width is only 2.8" wider than the bottom.
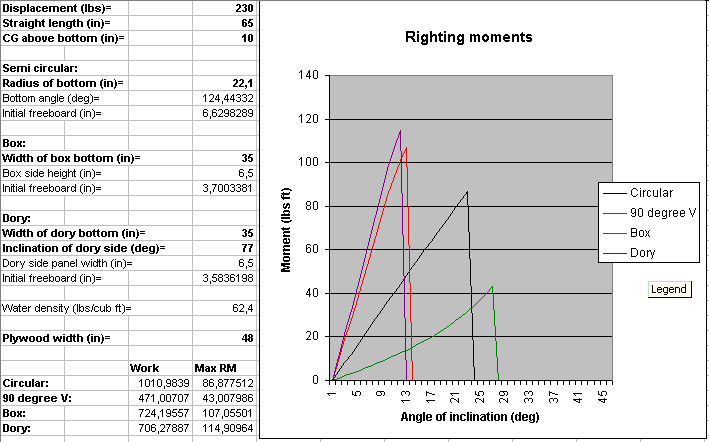
And then the maximum righting moments. The box shapes rule. But alas! Totally unacceptable initial freeboards! That should have been clear from the previous graph.
The only one with an acceptable freeboard is the semi circular.
All in all, here are some good shapes.
These shapes seem to give consistently reasonable, if not good, righting moment and work results over reasonable ranges of center of gravity heights.
But there's a lot of possible playing around. So try it out Yourself!